One sample Wilcoxon signed rank test in Excel tutorial
This tutorial explains how to run and interpret a one-sample Wilcoxon signed rank test in Excel using the software XLSTAT.
Dataset to perform a one-sample Wilcoxon signed rank test
The data represents the liking scores for a new crisps brand rated from 1 to 10 by 30 consumers. The median liking score of the strongest brand in the market is known to be 7.
Our goal, here, is to determine whether the median liking score of the new brand is higher than 7. For this purpose, we will perform a one-sample Wilcoxon signed rank test. This test allows the comparison of an observed median to a known standard value without assuming normality. It is a non-parametric alternative to the one-sample t-test.
The results of this test can indicate us whether consumers are globally more satisfied with the new product.
Setting up a one-sample Wilcoxon signed rank test with XLSTAT
Once XLSTAT is open, select the XLSTAT / Nonparametric tests / One sample Wilcoxon Signed Rank test as shown below**.**
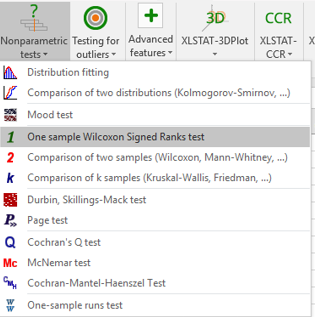

Once you have clicked on the function, the next dialog box appears:
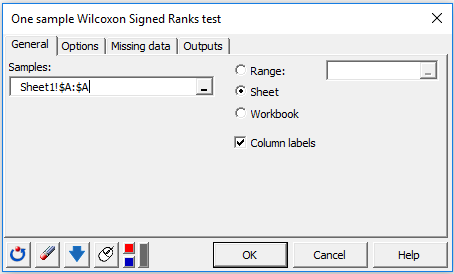
Select the liking scores from column A in the Sample field.
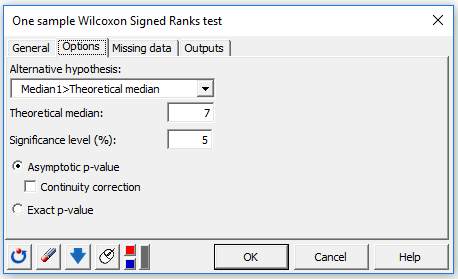
In the Options tab, choose Median1>Theoretical median as the alternative hypothesis and set the Theoretical median equal to 7. Given the selected hypothesis, the test will be an upper-tailed test (or right-tailed test).
Finally, we choose to compute the p-value using the asymptotic approximation of the distribution of the V statistic.
After clicking OK, the results are displayed on a new sheet.
Interpreting the results of a one-sample Wilcoxon signed rank test in XLSTAT
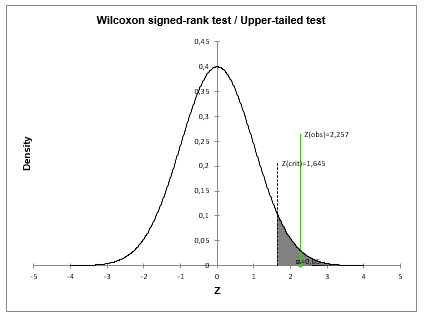
The following table presents the results of the upper-tailed Wilcoxon signed-rank test.
The computed p-value is lower than the significance level = 0.05 thus we can reject Ho and accept that the median of our sample is higher than 7. Another way to draw this conclusion is using the Z statistic. Here, Z = 2.257 is higher than the critical value at a 5% level of significance (1.645) so we can reject Ho. This can be easily observed in the following graph.
Was this article useful?
- Yes
- No
